The Angle on Pool

Resource for Grades 8-12

| Citation

Media Type:
Video

Running Time: 4m 52s
Size: 18.1 MB

Source: Learning Math: Measurement: "The Angle on Pool"

This asset is adapted from a video in Annenberg Learner's Learning Math: Measurement, a professional development course for teachers.

Resource Produced by:

Collection Developed by:

Collection Credits

Collection Funded by:

Related Resources:

Laser Waterfall
(Video)

Light and the Law of Reflection
(Video)

In this video segment adapted from Annenberg Learner’s Learning Math: Measurement, learn how players think about angles in the game of pool. See how you can plan ahead for the next shot by controlling the angle at which the cue ball bounces off the target ball. Discover how when the cue ball travels without spin, its rebound path is always perpendicular to the path of the target ball. See how a player can change the angle to be more or less than 90 degrees by hitting the cue ball with reverse or forward spin. In addition, learn how the law of reflection predicts the path of a bank shot (when a ball is purposely bounced off the rail): the angle of incidence of the ball will be equal to the angle of reflection after it bounces off the rail.

Permitted use:

Download and Share

Accessibility Features: Caption

Background Essay

Pool, or pocket billiards, is a game of mathematics and physics. The basic goal of play is to deposit balls into the pockets by hitting them with the cue ball. How the player strikes the cue ball (how hard, where, and in which direction) determines how the cue ball will interact with the other balls. The player can also purposely hit a ball into a rail of the table to have the ball bounce off and travel in a particular direction; this is called a bank shot. A skilled player understands how to control the path of the balls.

When playing pool, an important concept to understand is the symmetry of angles, as governed by the law of reflection. The law of reflection states that the angle of incidence—the angle formed by the path of the incoming object and the normal line (the perpendicular line) to the reflecting surface—is equal to the angle of reflection—the angle formed by the normal to the surface and the path of the object as it leaves the surface. According to the law of reflection, the angle at which an object hits a flat surface will be equal to the angle at which it bounces off the surface.

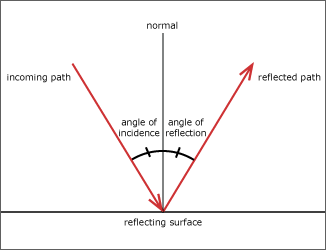
Law of Reflection Illustration

Assuming there is no spin on a pool ball and conditions are ideal (for example, the balls are perfectly round and the surface of the table is perfectly flat and frictionless), the angle at which a ball hits the rail of the table will be equal to the angle at which it rebounds. The symmetry of incident and reflected angles can be used to predict the behavior of pool balls as well as other things, such as how light bounces off a mirror or how sound bounces off walls.

In the game of pool, it is also important to consider the angle at which the cue ball rebounds off the target ball. Again, assuming ideal conditions and no spin on the cue ball, the cue ball will always rebound at 90 degrees from the path of the target ball (unless the collision is head-on). This "90-degree rule" is a result of physics—because momentum is conserved, after the collision, the cue ball must move in a direction that is perpendicular to the motion of the other ball.

Hitting the cue ball below or above its center will cause it to spin, which affects the angle at which it will rebound. By manipulating the spin of the cue ball, you can alter its rebound path to set it up for another good shot.

Printer Friendly Version of Background Essay

Print Background Essay

Connections

Connections to the Common Core State Standards

High School: Geometry

Congruence
- Experiment with transformations in the plane.
  - G.CO.4. Develop definitions of rotations, reflections, and translations in terms of angles, circles, perpendicular lines, parallel lines, and line segments.
Understand congruence in terms of rigid motions.
- G.CO.6. Use geometric descriptions of rigid motions to transform figures and to predict the effect of a given rigid motion on a given figure; given two figures, use the definition of congruence in terms of rigid motions to decide if they are congruent.

Grade 8

Geometry
- Understand congruence and similarity using physical models, transparencies, or geometry software.
  - 8.G.1. Verify experimentally the properties of rotations, reflections, and translations.
    - Lines are taken to lines, and line segments to line segments of the same length.
    - Angles are taken to angles of the same measure.

Print Connections

Teaching Tips

After the video

Have students discuss how they would use the law of reflection to help determine the point on the rail to hit for a bank shot. Draw a scenario on the board showing a rectangular pool table, a target ball placed near a pocket, and a cue ball. Have them draw lines on the board to help them figure out where the cue ball should bounce off the rail in order to hit the target ball into the pocket.
Have students prove geometrically or algebraically why the cue ball's path after hitting the rail is symmetrical to its incoming path.
Discuss how the 90-degree angle on the table would look different from certain perspectives. Have students use the board to draw a bird's-eye view of the 90-degree angle created by the pool balls. Ask students to consider how the same shot would look from various positions, such as:
- Eye level with the table
- Standing eye level (seen in the video)
If you have access to a pool table, have students work in small groups to demonstrate the 90-degree rule and the law of reflection. Encourage them to think through the angles carefully before taking a bank shot. You may want to have them cover the balls in chalk so that the tracks will be visible after each hit. They can then measure the angles with a protractor.